TDCC Laboratory
| HOME | JAPANESE |
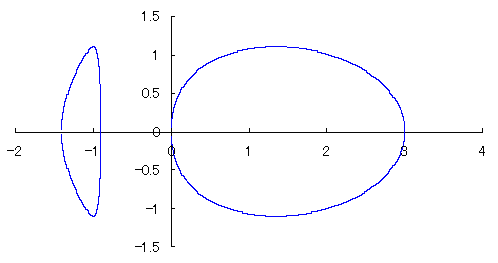
Egg Shaped Curve VII (Egg Shaped Curve III proposed by Mr. Asai)
Mr. Yasuyuki ASAI (Machida city, Tokyo, Japan) and Nobuo YAMAMOTO
Mr. Yasuyuki ASAI has informed novel type of an egg shaped curve to Yamamoto in April, 2011.
This report is described by YAMAMOTO in account of the equation of the egg shaped curve proposed by him,
|
The equation expressing a novel type of egg shaped curve
(obtained by the method using the difference between the first and the second order equations)
proposed by Mr. Yasuyuki ASAI is written in the following;
|
|
|
|
|
|

|
|
|
_ returning to the page which opened just before with 'return' of browser _
or
_ returning to the HOME with the following button _
| HOME |
 ,
(1)
,
(1)
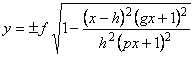 .
(3)
.
(3)
 .
(4)
.
(4)
 ,
(6)
,
(6)